Back • Return Home
Infinity In A Wildflower
This is a based on an email exchange with a friend from Feb. 2022. It will serve as a placeholder for a future article on this topic...
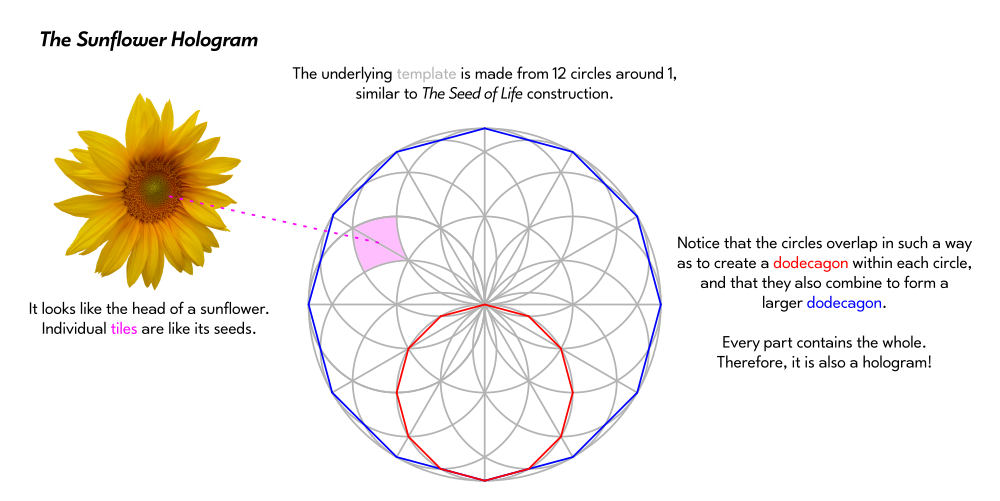
The pole view of The ABHA Torus is referred to as The Sunflower Hologram. It looks something like this:
I've used 12 circles to make the pattern easy to understand, but the actual construction uses 36 circles (or 12 × 3).
When the numbers are shown within each tile of The Sunflower Hologram, it can function like a type of log-polar coordinate system that greatly simplifies Trigonometry. In other words, trigonometric functions with endless decimals can be given whole number representations using Mod-9. This is based on two facts: That 0.999... = 1 and that 360 is a multiple of 9. There is also a relationship between The Unit Circle and Pythagorean Triples that plays a role here.
A similar thing can be done with Pi and other "real numbers". This is why the ancients used the "Diophantine approximation" of 22/7. Notice that it is equivalent to 3 and 1/7. When we divide out 1/7 we get the repeating "Ennegram" pattern of 0.142857...
There are actually quite a few applications of this to Physics and Biology, but applying this type of math to computing would allow us to do a type of "fractal compression", which can reduce complex files to tiny algorithms that take up only a fraction of the original space. The closest thing that I've seen to this is the image processing work of Carl Weiman and George Chaikin. They use a "logarithmic spiral grid" that is similar to how the photoreceptors in the retina are orgranized. It is essentially equivalent to the pattern that the tiles on the pole view of The ABHA Torus are making!