Back • Return Home
Ancient Egypt's Theory of Everything
Part 2: The Pyramids as Cosmic Temples
Robert Campbell, 2008
The First Old Kingdom Pyramids
Working with stone became an Egyptian specialty early in the dynastic period. The First Dynasty Pharaoh Menes is thought to have united upper and lower Egypt about 3100 BC and the capital was established at Memphis a short distance south of Cairo. A huge stone wall was erected around the city to protect it from the annual flood and this must have resulted in skills working with stone, using nothing more sophisticated than copper tools.
The first major pyramid was engineered by Imhotep for the Third Dynasty Pharaoh Djoser. An extensive underground funerary complex was hewn out of bedrock first and then the pyramid was erected over it. It is believed to have started as a stone mastaba structure above but was progressively extended in size and upward in six steps until it became the massive Step Pyramid reaching a height of 60 meters.
Djoser's successor Sekhemkhet left an unfinished step pyramid complex nearby. It may be that six steps were significant because six was regarded as a perfect number. It is both the sum and the product of its factors. We inherit our measure of circles and the cycles of time from the Mesopotamian “soss” for 60. Thus we have 60 seconds in a minute, 60 minutes in an hour, 6x60 degrees in a circle and so on. The numbers 6 and 10 were seen as interrelated in Egypt as well as in Mesopotamia, but it took on a different flavor in Egypt as we shall see.
Another step pyramid at Meidum was covered in a casing to give it a smooth pyramidal shape, which later fell away. It is usually attributed to Huni, the last king of the Third Dynasty but it may have belonged to or been completed by Sneferu his son, since there are no identifying inscriptions. Sneferu built two more pyramids, the Bent Pyramid and the Red Pyramid, the latter achieving a true pyramid shape. If it was a strictly a funerary objective one wonders why he needed two or perhaps three pyramids. He was the father of Khufu (Cheops) who built the Great Pyramid.
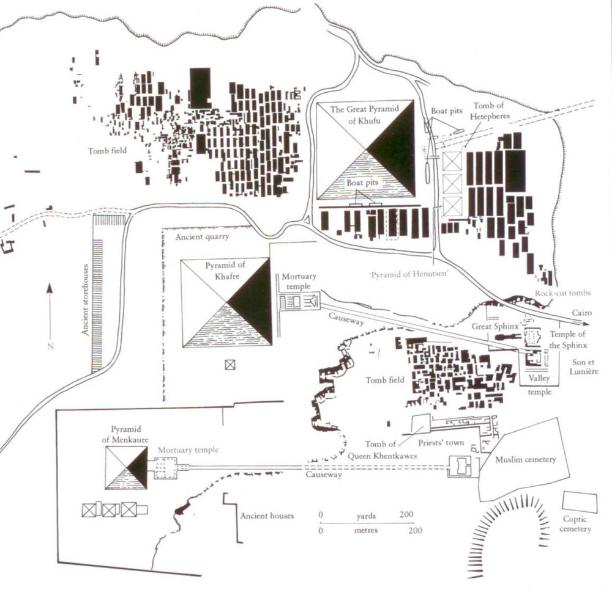
The Pyramid Complex at Giza and the Great Pyramid
There are indications that whole pyramid complex at Giza had a prior overall plan to it. An Idea gained momentum with Sneferu that took three succeeding generations of kings to complete. The first to be built and the most impressive was the Great Pyramid of Khufu, although the pyramid of his son Khafra is almost as large. That of Khafra's son Menkaura, although smaller is also impressive.
There are six so-called Queen’s pyramids that although smaller are still massive, with burial chambers inside. For example the largest near Menkaura's pyramid was originally 28.4 meters high, (93 feet) the other two being somewhat smaller. Three of them are aligned in a row immediately south of Menkaura's pyramid, and three are aligned in a row immediately east of Khufu's Pyramid. The three beside Khufu's pyramid are larger. So there are nine pyramids in all. Or should that be ten, because there is a small single pyramid immediately south of Khafra's pyramid. Is there some significance to all this? We will come back to this question later.
Sir Flinders Petrie conducted the first very precise survey of the pyramids between 1880 and 1882. His work is still used as a standard reference. His book "The Pyramids and Temples of Gizeh" was published in 1883 and can be accessed freely on the net. He also evaluates various theories that had arisen, discrediting most, confirming others.
Petrie determined that the accuracy of the Great Pyramids workmanship is such that the four sides have a mean error of only 58 mm in length and 1 minute in angle from a perfect square. The sides are closely aligned to the four cardinal compass points to within 3 minutes of arc based not on magnetic north but on true north. Continental drift or the precession of the poles might account for this small discrepancy. The original design dimensions, as confirmed by Petrie's survey and all that followed, were 280 royal cubits in height by 4x440 royal cubits around. (One royal cubit was 0.524 meters.) As these proportions equate to 2 x Pi to an accuracy of better than 0.05% this was and is considered to have been the deliberate design proportions. The height of the pyramid was the radius of a circle whose perimeter was equivalent to the perimeter of the base.
There was an empty sarcophagus found in the king's chamber.
The Great Pyramid was the world's tallest building for 4,000 years. It was originally 146.6 meters or 480.9 feet tall. Each base side was 440 x 0.524 = 230.56 meters or 755.8 feet. There were over 2.4 million stones averaging about two tons. To build it in 20 years meant quarrying, shaping, hauling and placing a stone every two minutes on average, with great accuracy. The organization required to accomplish such a feat within Khufu's lifetime was itself remarkable.
Khafra's Pyramid
Khufu's son, Khafra's pyramid was also built to very exacting standards. The pyramid has a base length of 215.25 m (706 ft) and originally rose to a height of 143.5 m (471 ft) The slope of the pyramid rises at an 53° 10' angle, steeper than its neighbor Khufu’s pyramid which has an angle of 51°50'40". Petrie confirms that this pyramid was built on the plan of a 3, 4, 5 triangle, the only right angled triangle with all three sides successive whole numbers. This too has significance which will be pointed out later.
The SW to NE diagonal axis of Khafra's pyramid is offset slightly from that of Khufu's pyramid although the diagonals and sides remain accurately parallel. This contrasts with the larger offset of Menkaura's pyramid which is also rotated slightly in its relative orientation. There is an interesting theory on this at www.legon.demon.co.uk that relates to the squaring of the circle.
There was a hiatus in building the Giza pyramids. Khufu had several sons and his immediate successor was his son Djedefre (Radjedef) who for some reason chose to build his pyramid at Abu Roash north of Giza. There is some speculation among scholars that there was a religious schism between elder and younger members of Khufu's successors. In any case Khafra again took up building at Giza eight years later when he became king after his half brother's death. There was an empty sarcophagus found in the main burial chamber hewn into bedrock beneath Khafra's pyramid.
Menkaura's Pyramid
Menkaura's pyramid was less accurate in its construction, although its original slope was estimated by Petrie to be 51°20'25", close to that of Khufu’s pyramid at 51°50'40". The bottom courses were capped with granite which remained rough cut on its outer edges, so that final surfacing was never completed. The upper courses were capped with limestone and apparently finished to present a smooth slope.
The main burial chamber was hewn into bedrock beneath the pyramid base. The sarchophagus was found empty with the lid missing, although pieces of the lid were found. Unlike those of Khufu and Khafra it was of ornate design, but it was unfortunately lost when the ship carrying it to Britain sank. Apart from this sarcophagus the Giza pyramids generally lacked any decor or inscriptions of any kind. This is in sharp contrast to later works and tombs.
The technology certainly existed to attain great accuracy in Menkaura's smaller pyramid, suggesting another factor in the overall plan. We will come back to this later.
The Divergence of Later Pyramids
Pyramid building continued off and on for about 2000 years but later pyramids were smaller and often shoddily built, lacking the accuracy and significance of the Giza complex. In general tomb building hewn out of bedrock took precedence over pyramid building and became characterized by extravagance as at the Valley of the Kings near Luxor. The tombs doubled as a storehouse of treasures to accompany the pharaoh in the afterlife. Elaborate décor portrayed the pharaohs as gods, a feature completely absent from the Great Pyramids at Giza.
Whether or not the Giza pyramids were intended as tombs, they certainly have obvious cosmological significance implicit in the stark simplicity of their geometrical design, lacking in hieroglyphics or internal décor. Indeed the only likeness of Khufu that has ever turned up is a 3" ivory carving bearing his cartouche. Petrie found it at the ancient site of Abydos, between Giza and Luxor (Thebes). Almost nothing is known of Khufu. There is none of the self aggrandizement that characterized the tombs of later periods.
An Overall Plan Spanning Four Generations
There must have been some incredible incentive, some driving impulse or insight that instigated such massive undertakings that strained the whole population of Egypt. The undertaking enlisted the cooperation and support of the whole populace. The Weighing of the Heart theme is obviously inconsistent with forced labor on such a scale.
It may or may not have begun with Imhotep, the builder of Djoser's Step Pyramid, which seems to have been an ad hoc affair that evolved during construction. It nevertheless could be indicative of insights that came as a staged development. Djoser's successor Sekhemkhet left an unfinished step pyramid complex nearby. There were no inscriptions apart from his name inscribed on the stoppers of clay jars found in the pyramid. When his still sealed sarcophagus was opened in the mid 20th century, it was found empty.
Sneferu (2613 to 2589 BC), the first king of the Fourth Dynasty, was certainly possessed of a compulsion to build pyramids, probably three in all. He passed his compulsion on to his son Khufu, who reached extraordinary heights. Then another two generations followed at Giza in what has the earmarks of an overall plan spanning four generations. There are specific relationships between the pyramids, their geometry, and their history, to clearly suggest this.
Squaring the Circle
The circle suggests a time-like dynamic principle whereas a straight line of fixed length suggests a static linear extension in space. Hence the tools of the sacred geometry were the ruler and compass. And one way to reconcile the two is to draw the square whose perimeter is the same as the perimeter of a circle. It is called squaring the circle.
There was an interesting book published by John Michell, City of Revelation, 1972. In it he explores many correspondences between esoteric subjects, from St. John’s revelation of the New Jerusalem, to the ground plan of Glastonbury Abbey, to Stonehenge, to the Great Pyramid, to gematria, to ancient systems of measure, and so on. Without going into the sense or reliability of all of them, a few salient features stand out as undeniable, and that deserve review.
A central theme of Michell's book is that there was a sacred geometry that was passed down through works in stone as a secret tradition of masonry. It may be connected to the establishment of the Masonic Lodge but the connection is lost in the mists of history somewhere in the Middle Ages along with the sacred geometry associated with it. Nevertheless the emblem of the Masonic Lodge is the ruler and compass.
There is a common feature of the creative process that does not make rational sense. If we draw a circle on a piece of paper the diameter of the circle can not be measured completely accurately in the same units used to measure the circumference, and vice versa, and yet the circle is clearly there on the page. For this reason the ratio between diameter and circumference, known as Pi, is called an irrational number. It has no completely discrete value. It goes on for ever and ever with no discernable pattern to the endless sequence of numbers. The diameter and circumference are said to be incommensurable. It is like the indeterminacy principle of quantum mechanics where the static position and dynamic momentum of a moving particle can never be known accurately together.
The Great Pyramid is thus a monumental example of squaring the circle, since its height is related to the perimeter of its base by the ratio 2 Pi. In this sense it is a monument that reconciles subjective spiritual dynamics to objective spatial regularities.
Squaring the circle is often dismissed by academics as simply impossible since Pi is an irrational number, but they miss the point. The point is that the linear regularities of space are reconciled with the cyclic dynamics of the heavens through living processes. And the heavens are dominated by circular motions. The universe is a throbbing living reality. That was the theme of the sacred geometry.
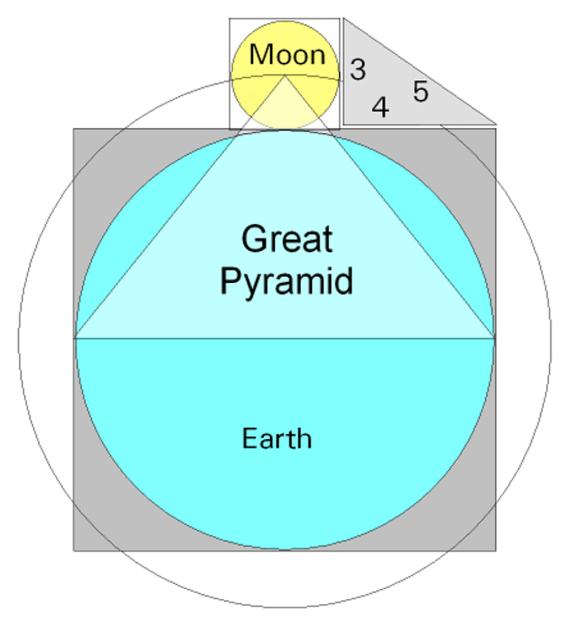
The Relationship of the Earth and Moon to the Khufu and Khafra Pyramids
As Michell points out, if we draw a scale diagram of the earth and moon so that they are touching, and enclose each in a square, a surprising relationship becomes obvious. It is illustrated in the diagram (borrowed from my book Fisherman's Guide.)
The triangle with apex at the center of the moon and base across the diameter of the earth has the same proportions as the Great Pyramid of Khufu. The circle scribed through the center of the moon concentric with the earth has a circumference equal to the square that contains the earth to an accuracy of 0.0588%. The earth radius of 6,378 km and moon radius of 1,738 km are taken from standard reference tables. They could be out by that much given the irregularities of the earth and moon surfaces. So the dimensions of Earth and Moon themselves represent squaring the circle. Also the triangle that joins the square of the moon to the square of the earth has sides in the ratio of 3, 4, and 5. This same ratio corresponds to the slope of Khafra’s pyramid as confirmed by Petrie according to his meticulous survey. Michell also points out that the two concentric circles are in the same proportion as the bluestone and sarsen circles of Stonehenge.
It is a remarkable coincidence that the actual dimensions of Earth and Moon correspond to squaring the circle. There are other remarkable coincidences that will be explored later.
The Nine Giza Pyramids and Fractals of Unity
Try a little test. Sketch four separate circles on a piece of paper. Now try and draw all of the ways that four circles can be arranged with respect to their inside and outside. For example one can be drawn inside one of the others but separate from the remaining two. Or the remaining two can also be drawn one inside the other but separate from the other two. Or the circles can be drawn as four concentric circles. And so on. How many ways are there? You will find that there are only nine possible ways to do it.
These nine ways that derive from four circles correspond to the nine Terms of System 4 at www.cosmic-mindreach.com. The circles represent active interfaces. They share a common active center inside and a common passive periphery outside. Because they share a common active center we can call them centers. How they relate to one another in each Term and between Terms outlines the basis of meaning. This System is introduced on the website. We will come back to this later.
All nine ways relate to the same four circles that are employed to explore a unifying principle of subjective to objective relationships. This is analogous to squaring the circle, since the circle represents the subjective animating principle inside living creatures as they relate to the linear regularities of objective experience outside. This self-similar analogy is apparent in the way the whole of experience is integrated. It is representative of the cosmic order.
Number systems thus also exhibit self-similar analogous patterns that are hierarchically subsumed. This means that arithmetic and mathematics in general derive from the way the cosmic order works, not vice versa.
The Egyptians assigned no symbol for zero although their number system was designed on the base ten. They had separate symbols for 1, 10, 100, 1,000, 10,000 100,000, and 1,000,000 and so they generally had no need for a symbol for zero. Their number system was based on progressive whole number powers of ten. In other words it was logarithmic to the base ten but only in terms of the whole number digits from 1 to 9. There are only nine repeated ones between 1 and 10. There are only nine repeated tens between 10 and 100. There are only nine repeated 100's between 100 and 1,000 and so on. The subsumed repetition of nine holistic elements of counting is essentially a fractal representation of Unity.
Egyptian Fractions as Fractals related to the Giza Pyramids
The fractal representation of unity extends to fractions. Egyptians allowed only unit fractions. They saw fractions as reciprocals of whole numbers. Their understanding of numbers, mathematics and measurements was implicitly holistic in nature and related to the cosmic order.
This brings us to another peculiarity of the numbers from 1 to 9. The reciprocals of the numbers 3, 6 and 9 repeat themselves to infinity. They yield the infinite numbers 0.3333etc., 0.16666etc., and 0.11111etc. The reciprocal of the number 7 is also an infinite number but it is the repeating sequence of the remaining six numbers, namely 0.142857142857142857etc.
The numbers from 1 to 9, apart from 7, when each divided by 7 also result in the same repeating six digit sequence but it starts at different places in the sequence. The number 7 divided by itself is Unity of course, so there is something about the reciprocal of 7 that regenerates the other numbers endlessly as they relate to unity. Numbers as fractals of unity proliferate as reciprocals of themselves and the number 7 represents the memory of the computational proliferation. In fact any number divided by 7 has decimal positions that endlessly repeat the same six digit sequence, unless the number is an exact multiple of 7. The Egyptians had a system of division that worked in such a way that they must have known this. They must also have known about irrational numbers that are incommensurable.
This is a further indication that the Giza pyramids represent the comic order in their stark geometry and in the overall plan. The obvious fact that there are three large pyramids and six small ones suggests a direct correspondence without getting into a lot of spurious speculation. The reciprocals of 3, 6 and 9 relate to the three large pyramids. The repeating six member sequence of 7 relates to the six small pyramids. The tiny tenth pyramid indicates the decimal system their numbers are based upon.
The Pyramids, the System and the Cosmic Order
There are direct parallels between the Giza pyramid complex and the System as introduced on the website. The System as an expression of the cosmic order consists of a nested hierarchy of subsumed higher Systems that all derive from requirements implicit in the nature of universal wholeness. Each higher system is a discrete elaboration of the lower systems that precede it. The lower systems thus transcend and subsume the higher systems such that the whole nested hierarchy is a subsumed elaboration of System 1 and universal wholeness. Each higher system is nevertheless complete unto itself as it relates to phenomenal experience. All possible structural varieties of experience are thus encompassed. It is to this extent a Theory of Everything. It is not possible to conceive of anything outside it. More accurately it is a Universal Methodology that must find consistency with the living empirical evidence much like Ancient Egypt’s living insights. It is not a Theory of Everything as an abstract construction distinct from a living reality such as the kind that science currently seeks.
In System 2 there are only two ways that two circles can be drawn with respect to inside and outside. One of the circles must be universal and unique and the other must be particular and many in order for System 2 to be a consistent elaboration of System 1 and universal wholeness. The two circles can be separate facing one another, or one circle can be inside the other. This implicitly defines both a subjective and an objective basis to experience.
In System 3 with three circles there are four possible ways to draw them with respect to inside and outside. Two of these Terms are universal and two are particular but they combine to work in pairs as an elaboration of System 2. The pairs alternate between an objective and a subjective orientation.
In System 4 with four circles there are nine ways to draw four circles, each called a Term, and they interact as an elaboration of System 3. Three of the terms are universal and six are particular. The six particular terms occur in alternating synchronous groups of three. One group has an objective orientation. The other group of three has a reciprocal subjective orientation. So one grouping is orthogonal or at right angles to the other, like the two groups of three small pyramids at Giza. The six particular terms also relate in a subjective to objective orientation as polar pairs that correspond to the three universal terms and the three large pyramids at Giza.
Summary
These observations together with the stark geometry of the Khufu and Khafra pyramids that correspond so accurately to the proportions of Earth and Moon make a powerful statement. These two largest pyramids indicate the reconciliation of the living subjective dynamics of the circle that we experience inside with the stark objective linearity of space and time that we experience outside. These two perspectives must find reconciliation through living processes.
Menkaura's pyramid did not have to be geometrically accurate to take its place as the third member of the infinite triad. In fact it is rotated slightly as if on purpose to set it apart for this reason. Its rotation together with its three orthogonal pyramids suggests a dynamic relationship between the subjective and objective orientations implicitly represented in the other two pyramids.
Taken also in the context of the Weighing of the Heart as described in Part 1, it is a statement that portrays life itself as the reconciliation of otherwise incommensurable elements between the living subjective dynamics and the static objective regularities of the cosmic order.